HISTORY
Johannes Kepler: The Pioneer of Planetary Motion
- Published On
July 1st, 2024
- Author
Syeda Natasha Hassan
Early Life and Education
Johannes Kepler was born on December 27, 1571, in Weil der Stadt, a small town in the Holy Roman Empire that is currently part of Germany. He was born into a disruptive family setting. His father was Heinrich Kepler, a mercenary who traveled widely and often left the young Johannes and his mother, Katharina Guldenmann. Katharina, the daughter of a local mayor, was known for her interest in herbal medicine and alleged witchcraft. Both of Kepler’s parents fueled his passion for astronomy; according to him, at the age of six, his mother took him to a hill to observe a comet, and at the age of nine, his father took him out one night under the stars to observe a lunar eclipse. Despite his family's financial position, he showed potential that was recognized and supported through his education.

The house, situated in the historic town of Weil der Stadt in southwestern Germany, is celebrated for its association with Kepler’s early life and his subsequent groundbreaking contributions to science. (Photo Credit: Wikipedia)
The Lutheran Church dominated Kepler's early education and thus trained him in religious conviction, which he would later combine with his work in science. He was sent to the seminary school at Maulbronn, where he developed an exceptional talent for mathematics. In 1589, Kepler entered the University of Tübingen on a scholarship, initially studying theology but soon becoming captivated by mathematics and astronomy under the mentorship of Michael Maestlin, a prominent astronomer who introduced him to the Copernican heliocentric model. This was despite his ambition to join the priesthood, as the prevailing Christian view at that time was that the Earth was the center of the universe. Later, in 1594, he was appointed the official instructor in astronomy and mathematics at the University of Graz in Austria, where he used most of the time for his own research.
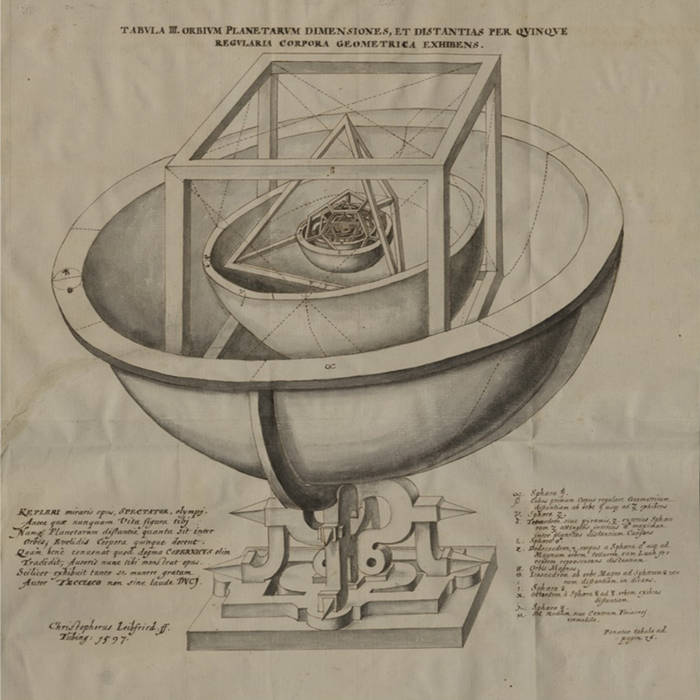
Taking leverage of his research, Kepler published his Mysterium Cosmographicum (The Cosmographical Mystery) in 1596 and expanded it in 1621. In this seminal book, Kepler proposes an innovative cosmological theory where the distances of the six known planets are elucidated through the geometric framework of the five Platonic solids—cube, tetrahedron, octahedron, dodecahedron, and icosahedron—each nested within a sphere representing planetary orbits. Kepler’s approach was revolutionary, suggesting that these geometrical shapes, which he believed to be divinely ordained, reflected a harmonious and divine structure of the universe. This model, emerging from Kepler's epiphany while demonstrating geometrical relationships, was one of the first serious attempts to support the heliocentric theory since Copernicus, offering a tangible, geometric rationale for planetary distances and reflecting Kepler's deep-seated belief in the unity of science and divine order.
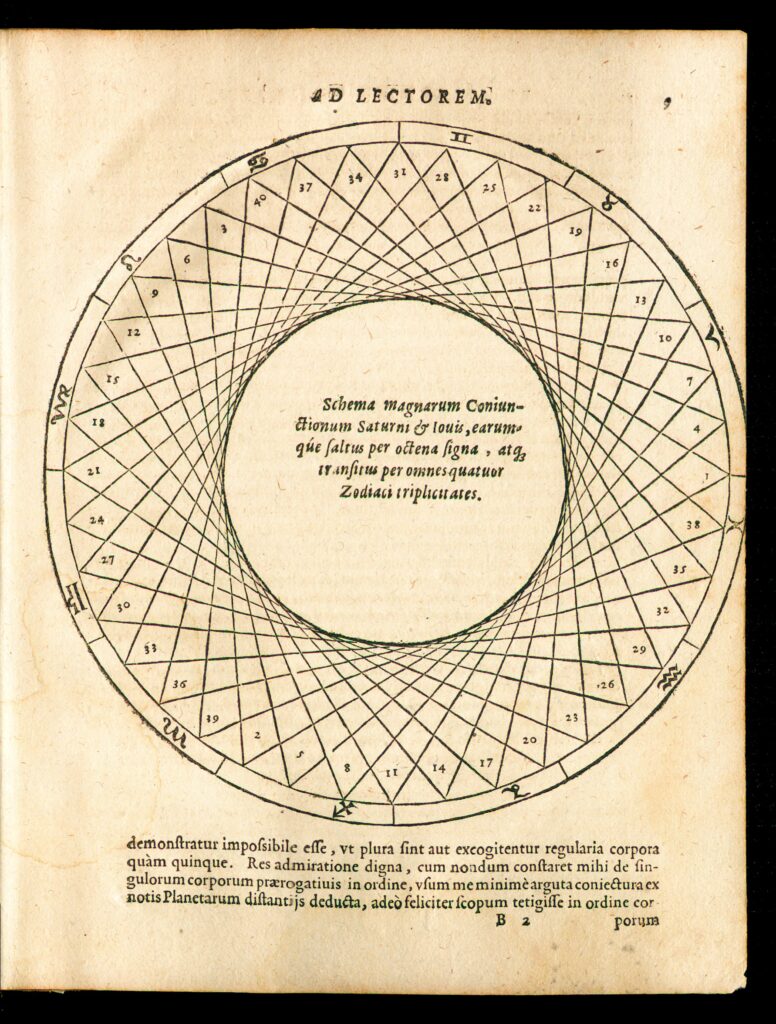
Illustration from Johannes Kepler's Mysterium Cosmographicum (1596) depicts the intricate movement of Jupiter and Saturn through the zodiac constellations, with symbols represented in the outer circle. (Photo Credit: Thinking3d UK)
Personal Life and Challenges
Johannes Kepler’s personal life, while often overshadowed by his scientific achievements, was marked by significant trials and transitions. His years in Prague, from 1600 to 1612, were relatively peaceful and scientifically prolific, during which he made notable advancements in astronomy and mathematics. Despite these successes, his life began to unravel in late 1611. The death of his seven-year-old son, who had reminded him of his own youth, was a particularly heavy blow. This personal tragedy was soon followed by the death of his wife, Barbara. Compounding these losses, Emperor Rudolf II, whose health was deteriorating, was forced to abdicate in favor of his brother Matthias. Unlike Rudolf, Matthias was less tolerant of Protestants, which forced Kepler to leave Prague. Before departing, Kepler had Barbara’s body moved to rest with their son and wrote a Latin epitaph for them.
Kepler’s personal life also reflected a blend of personal devotion and practical necessity. His marriage to Barbara was arranged through a broker but was grounded in affection. In contrast, his second marriage in 1613 to Susanna Reuttinger was driven by practical needs; Kepler required someone to care for his surviving children. Susanna’s introduction to Kepler’s character was abrupt; during their wedding celebrations, Kepler’s curiosity about the measurement of wine barrels led him to explore volumes of solids of revolution. This investigation resulted in his 1615 work Nova stereometria doliorum ("New Stereometry of Wine Barrels"), where he applied methods of resolution into 'indivisibles' inspired by Archimedes.
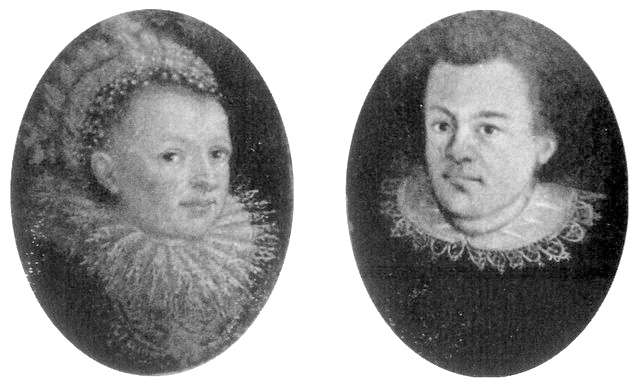
The early 17th-century portrait captures Kepler, renowned for his pioneering laws of planetary motion, alongside Barbara, highlighting their personal connection and shared life during a period of groundbreaking scientific discovery. (Photo Credit: Wikipedia)
Kepler’s unconventional ideas and Protestant faith frequently kept him out of favor with the Catholic Church and political powers, forcing him to move often and remain insecure about his employment. The strains of the Thirty Years' War, one of the most destructive conflicts in European history, and its associated social upheavals further complicated his life. His quest for knowledge often came at a great personal cost. For instance, his work Somnium, regarded as the first science fiction story, revealed his imaginative approach to science and his vision of celestial mechanics. Although he sought to return to a post at the University of Tübingen, his progressive theological views and affiliations with both Calvinists and Catholics met resistance from the faculty, reflecting his independent stance on religious matters. Consequently, Kepler was appointed to a newly created position as district mathematician in Linz while continuing his role as imperial mathematician under the new emperor, Matthias. Additionally, the Counter-Reformation’s impact in 1625 led to the temporary confiscation of his library by Catholic authorities and mandated that his surviving children attend mass, further complicating his already troubled personal life.
Major Contributions to Astronomy
Kepler's most influential contributions to astronomy are encapsulated in his three laws of planetary motion, which fundamentally transformed the understanding of the solar system. These laws were first presented in his groundbreaking work Astronomia Nova (The New Astronomy) in 1609, where he detailed the elliptical orbits of planets, challenging the previously accepted circular orbits. He further refined and expanded his theories in De Harmonices Mundi (Harmonies of the World) published in 1619, where he introduced his second and third laws, which describe the areas swept out by planets in their orbits and the relationship between a planet's orbital period and its distance from the Sun. These are the three laws in detail:
Kepler was the first to realize that orbits are better described by ellipses traversed at non-uniform speed than by combinations of 'perfect' circular motions on which orbits had hitherto been modelled. (Photo Credit: Thumos)
First Law (The Law of Ellipses):
Kepler stated that the planets move in elliptical orbits with the Sun at one of the foci. This means that the distance between a planet and the Sun constantly changes as the planet travels along its orbit. This groundbreaking discovery not only overturned the centuries-old belief in circular orbits but also profoundly transformed astronomical thinking by providing a more accurate description of planetary motion.
Second Law (The Law of Equal Areas):
He noticed that the line joining any planet's position with that of the Sun, in the course of motion, sweeps out equal areas during any equal intervals of time. This explained the variable speeds of planets that are faster near the Sun and slow down when away from the Sun.
Third Law (The Law of Harmonies):
Essentially, it states that the square of a planet's orbital period is proportional to the cube of the semi-major axis of its orbit. This law provides an exact mathematical relationship between the distances of planets from the Sun and their orbital periods. Specifically, it means that if you know a planet's distance from the Sun, you can calculate its orbital period with great precision.
Knowledge of Kepler's laws, particularly the second law (the law of areas), proved crucial to Sir Isaac Newton in 1684–85 when he formulated his famous law of gravitation. Newton extended Kepler's insights to develop a universal law that applied to all objects in the universe, not just celestial bodies. He demonstrated that while the motion of bodies subject to central gravitational forces typically follows elliptical orbits as described by Kepler’s first law, the paths can also be parabolic or hyperbolic depending on the object's total energy. This means that objects with sufficient energy, such as comets, can enter the solar system and leave it without returning. Additionally, Kepler’s second law implies that the angular momentum of a planet around an axis through the Sun and perpendicular to the orbital plane remains constant.
Brian Greene, in his Daily Equation series, illustrates how Newton’s law of gravitation governs the trajectories of planets and aligns with the patterns observed by Kepler. Kepler's laws extend beyond planetary orbits to the motions of natural and artificial satellites, stellar systems, and extrasolar planets. However, Kepler’s original formulation does not account for the gravitational perturbations between multiple planets. The challenge of accurately predicting the motions of more than two bodies under mutual gravitational attraction is complex, with analytical solutions to the three-body problem being rare. Kepler’s laws are also applicable to other inverse-square-law forces and, with adjustments for relativistic and quantum effects, to electromagnetic forces within atoms.
Calendar and Comets
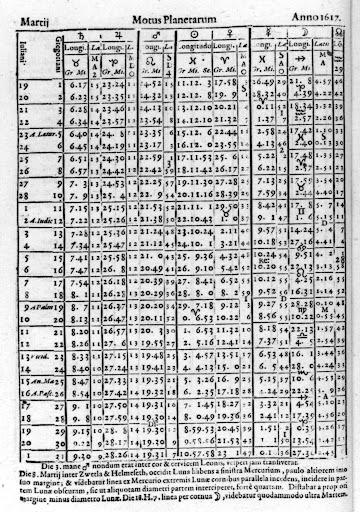
This table is from Johannes Kepler's Ephemerides, a key astronomical work in which Kepler meticulously charted the positions of celestial bodies over time. The table provides detailed calculations and predictions about the movement of planets, showcasing Kepler’s innovative approach to tracking planetary motion and his contribution to the field of astronomy. (Photo Credit: Department of History and Philosophy of Science)
Although Kepler’s earlier works were largely based on long-established plans, his calendars from 1617 to 1624 reflect the restlessness and turbulence of his era. These six calendars not only forecasted future weather but also integrated Kepler’s moral reflections, illustrating his tendency to blend astrological insights with ethical considerations. For example, his 1618 calendar predicted significant celestial and terrestrial events, including the comets that appeared later that year. His predictions proved notably accurate, as evidenced by his foresight of the Prague defenestration in May 1618, which marked the beginning of the Thirty Years' War. The popularity of his calendars was high; the 1619 edition sold out within eight days, highlighting public interest in his forecasts.
In addition to weather predictions, Kepler used his calendars to address the broader implications of celestial events. He discussed the potential impact of comets and other astronomical phenomena on human affairs and natural events. Despite his scientific approach, Kepler also engaged in contemporary debates about the significance of comets, defending his views against critics such as Scipio Chiaramonti and Galileo. His diligent efforts during this period resulted in extensive revisions of his earlier works and a rich body of scholarly contributions, reflecting his unwavering commitment to understanding the cosmos amidst the challenges of his time.
The “War” With Mars
A defining aspect of Kepler’s work was his intense and laborious study of Mars, a process he referred to as his "War with Mars." Tycho Brahe, who had previously held the position of Imperial Mathematician, had amassed a wealth of observational data on Mars, but his focus was not on the intricacies of planetary orbits. After Tycho's death in 1601, Kepler inherited this extensive dataset and set out to make sense of it. Traditional models of planetary motion relied on circular orbits, which were insufficient to account for the detailed observations Tycho had collected.
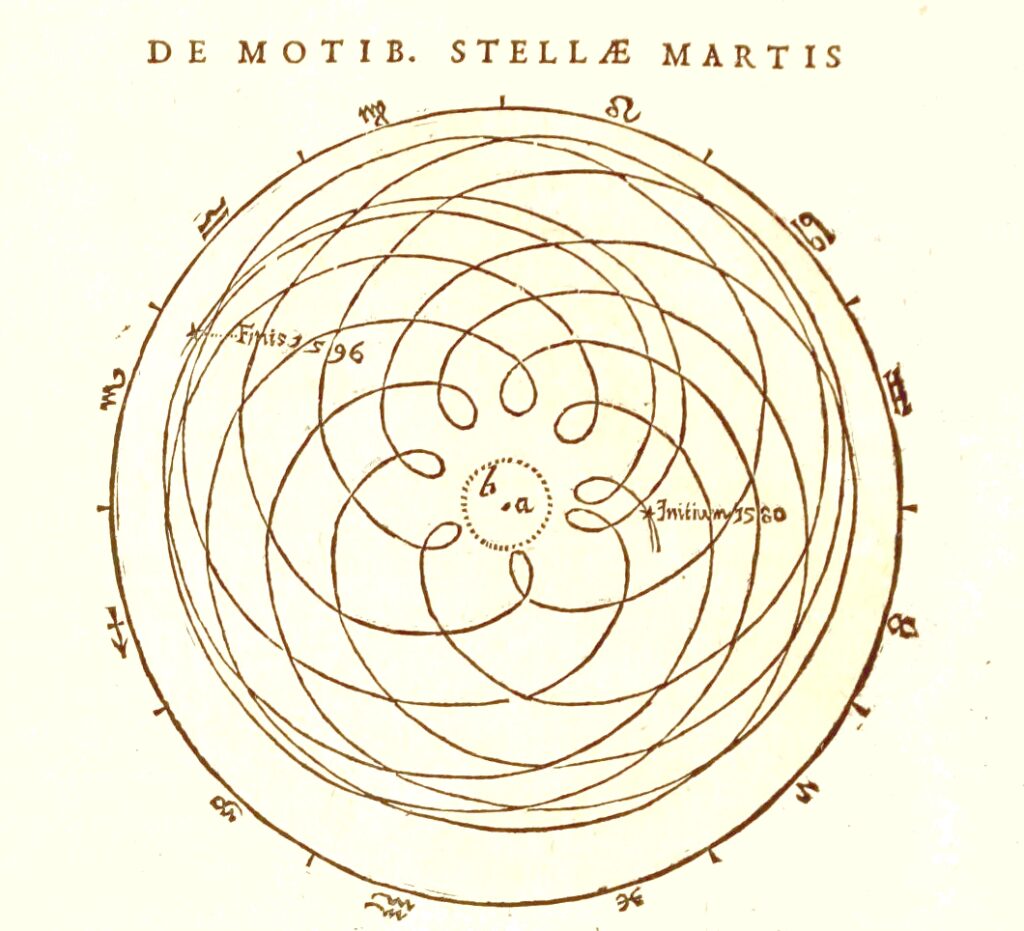
Johannes Kepler's depiction of the orbit of Mars, from his seminal work Astronomia Nova (1609), illustrates his revolutionary insights into planetary motion.
Kepler was determined to utilize this data to its fullest extent. He meticulously analyzed the observations, which involved nearly a thousand surviving folio sheets of arithmetic, to find a model that accurately described Mars’ motion. This exhaustive effort led to the realization that Mars' orbit was an ellipse with the Sun at one focus, a conclusion that would become known as Kepler's First Law. He also discovered that a line joining the planet to the Sun sweeps out equal areas in equal times, now known as Kepler’s Second Law. These findings were pivotal not only for Mars but were later extended to other planets, demonstrating that the same principles applied across the solar system.
Kepler’s painstaking work on Mars was a testament to his perseverance and ingenuity. The orbital model he developed matched modern results with such precision that comparisons must account for secular changes in the orbit since Kepler’s time. His ability to interpret Tycho’s data revolutionized our understanding of planetary motion and laid the groundwork for future advancements in astronomy. The success of these laws was further solidified when Kepler extended these principles to other planets, thus establishing a universal framework for celestial mechanics.
The Rudolphine Tables
Another monumental contribution to astronomy, in addition to his laws of planetary motion, was Kepler's work on the Rudolphine Tables. Named in honor of Kaiser Rudolf II, these tables were based on the meticulous observational data collected by Tycho Brahe, whom Kepler succeeded as the Imperial Mathematician. Tycho had invited Kepler to join his court at Castle Benátky near Prague. During his lifetime, Tycho had been stingy in sharing his observations. After his death (he passed due to a bladder ailment), although there was a political struggle with Tycho’s heirs, Kepler was ultimately able to work with data accurate to within 2′ of arc. Without data of such precision to back up his solar hypothesis, Kepler would have been unable to discover his “first law” (1605), that Mars moves in an elliptical orbit. Published in 1627, the Rudolphine Tables provided the most accurate planetary positions of their time, which significantly aided navigators and astronomers in their work. This achievement solidified Kepler's reputation as one of the great astronomers.

The frontispiece of the Rudolphine Tables honors the great astronomers of history, including Aratus, Hipparchus, Copernicus, Ptolemy, and Meton, with a special emphasis on Tycho Brahe, whose contributions were pivotal to the work. (Photo Credit: Britannica)
The Rudolphine Tables were accurate to within a few minutes of arc and included positions for 1,005 stars, an increase from Tycho’s original 777 stars. They also featured detailed tables and instructions for locating the planets. Notably, the Rudolphine Tables were the first catalog to incorporate corrective factors for atmospheric refraction, as well as logarithmic tables, enhancing their utility and precision. This work not only advanced the field of astronomy but also marked a significant improvement in observational accuracy, setting a new standard for future astronomical research.
Contributions to Optics
During the early 17th century, Johannes Kepler made groundbreaking contributions to the field of optics that profoundly influenced the understanding of light and vision. His work in this area was closely tied to his astronomical observations, particularly regarding how light behaves as it travels through different media. Kepler’s primary focus was on how light rays from distant celestial bodies are deflected as they enter the Earth’s atmosphere and subsequently interact with the human eye. This line of inquiry was not entirely new, as there were some medieval precedents, but Kepler approached these problems with innovative methods and fresh perspectives.
In 1604, Kepler published Ad Vitellionem Paralipomena, also known as Supplement to Witelo, in Which Is Expounded the Optical Part of Astronomy. This treatise was a significant departure from medieval optics, which had been largely influenced by the works of Witelo. Kepler’s analysis of vision fundamentally altered the framework for understanding light. He introduced the concept that every point on a luminous body emits light rays in all directions, but only the rays that strike the pupil of the eye are perceived. This insight led him to revise the traditional visual cone model, proposing instead that rays from a single luminous point form a cone with its base on the retina. This approach explained that the retina, as the sensitive receptor of the eye, is where light rays converge to form an inverted image.
Unlike modern telescopes, the Copernican model used a basic lens system to magnify distant planets and stars, contributing to the validation and refinement of the heliocentric model proposed by Copernicus. (Photo Credit: Wikimedia Commons)
Kepler’s theories extended to explaining the functionality of eyeglasses, which had been used for centuries but whose effectiveness lacked a clear theoretical basis. By providing a detailed explanation of how light rays are refracted within the eye, Kepler’s work offered the first comprehensive theory of why eyeglasses improve vision. His work revealed that if the eye’s optics were not functioning properly, the converging light rays would not focus precisely on the retina, resulting in blurred vision. This novel understanding of optical phenomena marked a significant advancement in both theoretical and practical optics. Due to these pioneering contributions, Johannes Kepler is often hailed as the "Father of Modern Optics." His innovative approach to the study of light and vision laid the foundation for future research and greatly advanced the field, making him a central figure in the development of optical science.
Legacy
The legacy of Johannes Kepler thus reaches much further than his times. Only through his accurate observations, mathematical stringency, and innovative thinking did astronomy turn into a precise science. His laws of planetary motion furnished the empirical foundation for Newton's theory of gravity and hence spanned an arch from Copernican heliocentrism to modern astrophysics. Kepler's work on optics laid essential groundwork for future developments, influencing scientists such as Christiaan Huygens, and helped develop the evolution of the telescope. In his interdisciplinary approach, combining mathematics, astronomy, and physics with philosophical and theological reflections, he displayed the Renaissance ideal of the polymath.
Due to these pioneering contributions, Johannes Kepler is often hailed as the "Father of Modern Optics." His innovative approach to the study of light and vision laid the foundation for future research and greatly advanced the field, making him a central figure in the development of optical science. Furthermore, Kepler's life and work underscore in bold the deep influence of perseverance and intellectual curiosity. Against overwhelming odds, Kepler never gave up on his discovery of the secrets of the universe, working from a sense of awe and faith in the harmony of creation. His contribution is where scientists and scholars find inspiration, reflecting on the spirit of inquiry and discovery that makes human civilization propel forward.
Conclusion
Johannes Kepler's life and works represent a significant part of the chain connecting science. So, his groundbreaking works on astronomy, optics, and mathematics changed the view of the universe, opening the way for forthcoming scientific revolutions. Kepler's legacy still shines as a beacon of human curiosity, acting on its perseverance, and the relentless pursuit of knowledge. As Carl Sagan eloquently put it,
“Kepler’s laws of planetary motion were the first great triumph of the new scientific method, and his work laid the foundation for the new astronomy of the future”
Subscribe To Our Newsletter
Receive amazing space news and stories that are hot off the press and ready to be read by thousands of people all around the world.
